Mathematical tracts on the lunar and planetary theories, the figure of the earth, precession and nutation, the calculus of variations, and the undulatory theory of optics.

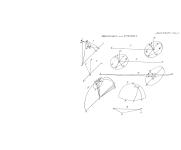
NEWTON'S SCALE OF COLOURS. 297:
(6) The transmitted light, just as in (68), produces
rings complementary to those produced by the reflected light. The center therefore is bright, and
is surrounded by a dark ring, which is followed by
bright and dark rings alternately, which soon become
coloured and finally cease to be visible. The diameter of each ring is the same as that of the ring
of opposite character produced by reflected light.
72. These are commonly called Newton's rings, from
the circumstance that the measures which suggested the
most important part of Sir Isaac Newton's theory of light,
and which have served in a great degree for the foundation
of all theories, were made by him on those rings. The
colours of the successive rings, arising from the different
mixtures of all the colours producing white light, are commonly called Newton's scale of colours. In describing
them it is usual to describe them by the number of the
ring (including the central spot in the reckoning) in which
they occur. For instance, the blue of the second order is
not the blue which surrounds the central black spot, but
the blue which surrounds the first black ring. This scale
is valuable, as giving us an invariable series of colours
which can at any time be produced without difficulty. The
colours described in (52) as resulting from the experiment
of Prop. 12 would do as well, but the adjustment of the
apparatus for that experiment is much more troublesome.
PitoP. 19. Light diverging from a center A, (fig. 20)
is allowed to pass through a small aperture BC: to find
the illumination on different points of the screen DE.
73. Suppose the wave diverging from A to proceed
in a spherical form till it reaches CB: there suppose every
part of it (within the limits of the aperture) to be the
origin of a little wave proportional in intensity to the superficial extent of that part. By the principle of (21), the
sum of the disturbances which each of these produces at
M is to be taken for the whole disturbance there: and
this being found, the intensity of light will be found as in