Elements of descriptive geometry, with applications to isometric projection and othe forms of one-plane projection; a text-book for colleges and ingineering schools by O. E. Randall.
Annotations Tools
RELATING TO POINT, LINE, AND PLANE 43 106. Problem 44. Draw the two projections of a straight line passing through the point l = - 4, - 4, 2, running parallel to V, and making an angle of 60 degrees with H. 107. Problem 45. Draw the two projections of a straight line passing through the point M=- 4, 3, -1, running parallel to H, and making an angle of 45 degrees with V. 108. Problem 46. Given the straight line [M= - 4, 5, 1; N= 4, 2, 6]; required to find the true distance between M and N, and to determine the angle which the line makes with H. Analysis. Revolve the line about an axis through M perpendicular to H until the line is parallel to V. The vertical projection of any portion of the line in this new position will be equal in length to the assumed portion of the line itself, and the angle which this vertical projection of the line in this new position makes with G-L will indicate the angle which the line itself in true position makes with H. 109. Problem 47. Given the straight line [Ai=- 4, 5, 1; N= 4, 2, 6]; required to find the true distance between the points M and N, and to determine the angle which the line makes with V. Analysis. Revolve the line about an axis through M perpendicular to V until the line is parallel to H. The horizontal projection of any portion of the line in this new position will be equal in length to the assumed portion of the line itself, and the angle which this horizontal projection makes with G-L will indicate the angle which the line itself in true position makes with V. 110. Problem 48. Draw the two projections of a straight line 5 units long, passing through the point M = 2, - 4, - 1, making an angle of 30 degrees with H, and in such a position that its horizontal projection makes an angle of 45 degrees with G-L. Analysis. First draw the projections of the line when it passes through the given point, is parallel to V, and makes the required angle with H. Then revolve the line about an axis through the given point perpendicular to H until the horizontal projection of the line makes the required angle with G-L. The two projections of the line in this last position will be the required projections,
-
Scan #1

Page #1
-
Scan #2

Page #2 - Title Page
-
Scan #3

Page #3
-
Scan #4

Page III
-
Scan #5

Page IV
-
Scan #6

Page #6 - Table of Contents
-
Scan #7

Page #7
-
Scan #8

Page 1
-
Scan #9

Page 2
-
Scan #10

Page 3
-
Scan #11

Page 4
-
Scan #12

Page 5
-
Scan #13

Page 6
-
Scan #14

Page 7
-
Scan #15

Page 8
-
Scan #16

Page 9
-
Scan #17

Page 10
-
Scan #18

Page 11
-
Scan #19

Page 12
-
Scan #20

Page 13
-
Scan #21

Page 14
-
Scan #22

Page 15
-
Scan #23

Page 16
-
Scan #24

Page 17
-
Scan #25

Page 18
-
Scan #26

Page 19
-
Scan #27

Page 20
-
Scan #28

Page 21
-
Scan #29

Page 22
-
Scan #30

Page 23
-
Scan #31

Page 24
-
Scan #32

Page 25
-
Scan #33

Page 26
-
Scan #34

Page 27
-
Scan #35

Page 28
-
Scan #36

Page 29
-
Scan #37

Page 30
-
Scan #38

Page 31
-
Scan #39

Page 32
-
Scan #40

Page 33
-
Scan #41

Page 34
-
Scan #42

Page 35
-
Scan #43

Page 36
-
Scan #44

Page 37
-
Scan #45

Page 38
-
Scan #46

Page 39
-
Scan #47

Page 40
-
Scan #48

Page 41
-
Scan #49

Page 42
-
Scan #50

Page 43
-
Scan #51

Page 44
-
Scan #52

Page 45
-
Scan #53

Page 46
-
Scan #54

Page 47
-
Scan #55

Page 48
-
Scan #56

Page 49
-
Scan #57

Page 50
-
Scan #58

Page 51
-
Scan #59

Page 52
-
Scan #60

Page 53
-
Scan #61

Page 54
-
Scan #62

Page 55
-
Scan #63

Page 56
-
Scan #64

Page 57
-
Scan #65

Page 58
-
Scan #66

Page 59
-
Scan #67

Page 60
-
Scan #68

Page 61
-
Scan #69

Page 62
-
Scan #70

Page 63
-
Scan #71

Page 64
-
Scan #72

Page 65
-
Scan #73

Page 66
-
Scan #74

Page 67
-
Scan #75

Page 68
-
Scan #76

Page 69
-
Scan #77

Page 70
-
Scan #78

Page 71
-
Scan #79

Page 72
-
Scan #80

Page 73
-
Scan #81

Page 74
-
Scan #82

Page 75
-
Scan #83

Page 76
-
Scan #84

Page 77
-
Scan #85

Page 78
-
Scan #86

Page 79
-
Scan #87

Page 80
-
Scan #88

Page 81
-
Scan #89

Page 82
-
Scan #90

Page 83
-
Scan #91

Page 84
-
Scan #92

Page 85
-
Scan #93

Page 86
-
Scan #94

Page 87
-
Scan #95

Page 88
-
Scan #96

Page 89
-
Scan #97

Page 90
-
Scan #98

Page 91
-
Scan #99

Page 92
-
Scan #100

Page 93
-
Scan #101

Page 94
-
Scan #102

Page 95
-
Scan #103

Page 96
-
Scan #104

Page 97
-
Scan #105

Page 98
-
Scan #106

Page 99
-
Scan #107

Page 100
-
Scan #108

Page 101
-
Scan #109

Page 102
-
Scan #110

Page 103
-
Scan #111

Page 104
-
Scan #112

Page 105
-
Scan #113

Page 106
-
Scan #114

Page 107
-
Scan #115

Page 108
-
Scan #116

Page 109
-
Scan #117

Page 110
-
Scan #118

Page 111
-
Scan #119

Page 112
-
Scan #120

Page 113
-
Scan #121

Page 114
-
Scan #122

Page 115
-
Scan #123

Page 116
-
Scan #124

Page 117
-
Scan #125

Page 118
-
Scan #126

Page 119
-
Scan #127

Page 120
-
Scan #128

Page 121
-
Scan #129

Page 122
-
Scan #130

Page 123
-
Scan #131

Page 124
-
Scan #132

Page 125
-
Scan #133

Page 126
-
Scan #134

Page 127
-
Scan #135

Page 128
-
Scan #136

Page 129
-
Scan #137

Page 130
-
Scan #138

Page 131
-
Scan #139

Page 132
-
Scan #140

Page 133
-
Scan #141

Page 134
-
Scan #142

Page 135
-
Scan #143

Page 136
-
Scan #144

Page 137
-
Scan #145

Page 138
-
Scan #146

Page 139
-
Scan #147

Page 140
-
Scan #148

Page 141
-
Scan #149

Page 142
-
Scan #150

Page 143
-
Scan #151

Page 144
-
Scan #152

Page 145
-
Scan #153

Page 146
-
Scan #154

Page 147
-
Scan #155

Page 148
-
Scan #156

Page 149
-
Scan #157

Page 150
-
Scan #158

Page 151
-
Scan #159

Page 152
-
Scan #160

Page 153
-
Scan #161

Page 154
-
Scan #162

Page 155
-
Scan #163

Page 156
-
Scan #164

Page 157
-
Scan #165

Page 158
-
Scan #166

Page 159
-
Scan #167

Page 160
-
Scan #168

Page 161
-
Scan #169

Page 162
-
Scan #170

Page 163
-
Scan #171

Page 164
-
Scan #172

Page 165
-
Scan #173

Page 166
-
Scan #174

Page 167
-
Scan #175

Page 168
-
Scan #176

Page 169
-
Scan #177

Page 170
-
Scan #178

Page 171
-
Scan #179

Page 172
-
Scan #180

Page 173
-
Scan #181

Page 174
-
Scan #182

Page 175
-
Scan #183

Page 176
-
Scan #184

Page 177
-
Scan #185

Page 178
-
Scan #186

Page 179
-
Scan #187

Page 180
-
Scan #188

Page 181
-
Scan #189

Page 182
-
Scan #190

Page 183
-
Scan #191

Page 184
-
Scan #192

Page 185
-
Scan #193

Page 186
-
Scan #194

Page 187
-
Scan #195

Page 188
-
Scan #196
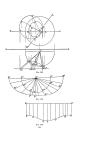
Page 189
-
Scan #197

Page 190
-
Scan #198

Page 191
-
Scan #199

Page 192
-
Scan #200

Page 193
-
Scan #201

Page 194
-
Scan #202

Page 195
-
Scan #203

Page 196
-
Scan #204

Page 197
-
Scan #205

Page 198
-
Scan #206

Page 199
-
Scan #207

Page 200
-
Scan #208

Page 201
-
Scan #209

Page 202
-
Scan #210

Page 203
-
Scan #211

Page 204
-
Scan #212

Page 205
-
Scan #213

Page 206
-
Scan #214

Page 207
-
Scan #215

Page 208
-
Scan #216

Page 209
-
Scan #217

Page #217
Actions
About this Item
- Title
- Elements of descriptive geometry, with applications to isometric projection and othe forms of one-plane projection; a text-book for colleges and ingineering schools by O. E. Randall.
- Author
- Randall, O. E. (Otis Everett), b. 1860.
- Canvas
- Page 34
- Publication
- Boston,: Ginn & company
- [c1905]
Technical Details
- Link to this Item
-
https://name.umdl.umich.edu/abn1872.0001.001
- Link to this scan
-
https://quod.lib.umich.edu/u/umhistmath/abn1872.0001.001/50
Rights and Permissions
The University of Michigan Library provides access to these materials for educational and research purposes. These materials are in the public domain in the United States. If you have questions about the collection, please contact Historical Mathematics Digital Collection Help at [email protected]. If you have concerns about the inclusion of an item in this collection, please contact Library Information Technology at [email protected].
DPLA Rights Statement: No Copyright - United States
Related Links
IIIF
- Manifest
-
https://quod.lib.umich.edu/cgi/t/text/api/manifest/umhistmath:abn1872.0001.001
Cite this Item
- Full citation
-
"Elements of descriptive geometry, with applications to isometric projection and othe forms of one-plane projection; a text-book for colleges and ingineering schools by O. E. Randall." In the digital collection University of Michigan Historical Math Collection. https://name.umdl.umich.edu/abn1872.0001.001. University of Michigan Library Digital Collections. Accessed April 29, 2025.