Coming of Age: Teaching and Learning Popular Music in Academia

Introduction

For the past few decades, the field of music theory has struggled with the question of how well traditional concepts—that is, those that were developed to describe and analyze the music of Bach, Beethoven, and Brahms—can be applied to popular music, for example, Beck, Björk, and Beyoncé. For instance, it is somewhat unclear if and how the precepts of classical functional harmony are relevant to rock music.[1] On the one hand, it seems useful to employ existing terminology when talking about new styles, if only because a common language helps us communicate and connect ideas. On the other hand, categorization schemes that were developed within a centuries-old musical practice might be ill-equipped to fully describe music of the modern era. Very often, the perfect balance between these two opposing views is difficult if not impossible to achieve.

Generally speaking, scholarship to date on pop/rock music—whether implicitly or explicitly—takes the use of traditional time signatures to be an adequate classification scheme for meter in pop/rock music.[2] Recent work focuses instead on analyses of metric dissonance, microtiming, and complex/mixed meters in specific styles (e.g., Butler 2001; Pieslak 2007; Osborn 2010; Danielsen 2010; McCandless 2013; Osborn 2014; Biamonte 2014), sidestepping issues of pedagogy and meter classification taken more broadly. One notable exception is Rosenberg (2011), who discusses the use of popular music in the teaching of rhythm and meter, but her goal is primarily to show how pop/rock songs can be used to illustrate traditional concepts, not how pop/rock songs might challenge them.

In contrast to this earlier work, I argue in this chapter that traditional time signatures are limited in their ability to fully represent the typical metric organizations found in pop/rock music, and accordingly, it is useful to include features beyond the traditional time signature in descriptions of rhythm and meter in pop/rock music. Specifically, I posit that two additional factors—swing and drum feel—are critical components of meter in pop/rock music and that our classification system should include information about these factors to adequately portray the rhythmic and metric hierarchy of a song. I thus do not deprecate traditional time signatures; rather, I show that the combination of traditional time signatures with various drum feels and swing rhythms engenders a robust language to catalog the diverse landscape of normative metric configurations found in pop/rock music. My focus here will thus be on a general scheme, and I will not address complex, additive, mixed, or irrational meters. (Theoretical investigation into these interesting but less common metric organizations can be found in the references cited in the previous paragraph.)

For some readers, the addition of categories beyond traditional time signatures might seem like the byproduct of an overactive concern with taxonomy. The conventional approach—by which a piece of music is considered, at least on a basic level, to be duple or triple (whether the beat is organized into groups of two or three) and as simple or compound (whether the beat divides into two or three)—is, one might argue, an elegantly efficient categorization scheme, one that balances too much versus too little information.[3] Indeed, it is entirely feasible to categorize meter in pop/rock with this basic scheme as a starting point. But as I show below, this approach ignores many important aspects of rhythmic and metric organization in pop/rock music. In adopting a more detailed language for meter in pop/rock, therefore, I hope to attune the reader (and thus our students) to these other central features.

As a sort of epistemological background, my approach to meter in pop/rock music derives from my own teaching of meter in the classroom. In my current position, I teach music theory in the department of recording industry, which is structured primarily around popular music; classical, jazz, and art music is taught in the music department across campus. For a number of semesters, I taught meter identification exclusively through traditional time signatures. This approach was manageable, although I began to sense that using this method was often like trying to fit a square peg into a round hole. I now teach using the approach I describe in the following sections, which arose in part through research on how popular musicians think about and talk about rhythm and meter. My bibliography, for example, includes many practical “how-to” books written by professional drummers and studio musicians (e.g., Matthews 1984; Morgenstein and Mattingly 1997; Randall and Peterson 1997; Potter 2001; Mattingly 2006; Zoro 2007; Riley 2010; Williams 2012; Zoro 2013; Riley 2015). I do not purport that my method mirrors how all pop/rock musicians conceptualize meter—certainly, there are many ways to approach these issues—but I believe I have developed a flexible approach that best balances both traditional and contemporary perspectives.

Before bringing this introduction to a close, I should mention that the current chapter serves as a sequel to the paper I gave at the Ann Arbor IV symposium, which was recently published in a longer version (2016). In that paper, I argue that pop/rock songs typically exhibit a fairly moderate pacing of harmonic and melodic content and that this pacing of harmonic and melodic content is relatively stable in terms of absolute time, despite the various rhythmic frameworks that might be implied by the drum pattern. Specifically, I argue that the optimal length for a measure is about two seconds, and so—all else being equal—we are often better guided when assessing measure lengths by this two-second ideal than by any particular drum pattern. In what follows, I consider the implications of that article for a classification system of rhythm and meter in pop/rock music. I recommend reading my 2016 article before engaging with the following discussion. That said, the current chapter does not necessarily require any familiarity with the earlier article, since I will touch on the more relevant aspects when necessary.

Drum Feels In 4/4

As many readers are probably aware, most pop/rock songs can be categorized as having a simple quadruple meter—that is, a time signature of 4/4.[4] Given a time signature of 4/4, the drum pattern of a song will often be organized according to the model shown in Figure 1, which I refer to as the “standard,” or “normal,” rock beat.[5] In a normal rock beat, the kick occurs on beats 1 and 3 of a 4/4 bar, while the snare occurs on beats 2 and 4. Drum patterns in real songs often depart from this exact configuration, of course, since the drummer will typically include a great amount of variety and embellishment around this basic framework. For example, the kick pattern might include smaller note values or anticipations of the beat, as shown in Figure 2, or the hi-hat pattern might consist of eighth notes instead of quarter notes.[6] But if we strip away the ornamentation in any real drum part, the model shown in Figure 1 will often be the underlying scheme. The widespread use of the standard rock beat across all styles of popular music—including rap, blues, country, folk, electronica, and R&B—has encouraged many contemporary scholars to use it as the primary determinant of measure lengths and time signatures in popular music.[7]

Using this standard rock beat as the universal yardstick for time signatures and measure lengths turns out to be a somewhat problematic strategy, however. In this regard, consider the song “Human Nature” by Michael Jackson (1982).[8] The reader should take the opportunity now to listen to this song, and while doing so, consider where the primary beat lies and how the drums interact with this beat. In my own hearing, the primary beat of “Human Nature” occurs around 92 beats per minute (BPM). Hearing the song this way, the kick and snare pattern does not conform to the standard rock beat; rather, it operates as what professional drummers refer to as a “half-time feel” (see Figure 3), whereby the snare regularly occurs on beat 3 instead of on beats 2 or 4, and the kick occurs only on the downbeat of the measure instead of on beats 1 and 3.[9] (For reference, the reader can hear a “normal-time feel” of “Human Nature”—in which the kick and snare align with the 92 BPM primary beat—in Tarrus Riley’s 2009 cover of the song.)

The fact that drummers often refer to the scenario shown in Figure 3 as a change in the drum “feel” rather than a change in the drum “pattern” relates, I believe, to the perceptual effect of shifting the metric location of the snare. When the snare is on beat 3, it “feels” as if the tempo is half as fast as if the snare were on beats 2 and 4, but for various other reasons (some of which I discuss in the following two paragraphs), the primary beat level is perceived at a different rate than what is implied by the alternation of the kick and snare. Without question, the overall rhythmic “feel” of the drums involves much more than the question of what particular level the snare articulates in the metric hierarchy. That said, in this chapter—so as to remain faithful to the vernacular terminology used by professional drummers—I use the term “feel” (e.g., different “drum feels”) to refer specifically to situations where the tempo implied by the drums (especially the backbeat as implied by the snare) might differ from or conflict with the primary beat level implied by the rest of the music.

I should concede that it is not impossible to hear the rate of kick and snare alternations in “Human Nature” as the primary beat itself, thereby engendering a tempo of around 46 BPM. I would speculate, however, that most listeners perceive the beat of this song as 92 BPM, if only because it is easier to dance to the song at this faster tempo.[10] As some evidence of this, note that the rhythmic body movements of Michael Jackson during various live performances currently available on YouTube tend to occur at the 92 BPM rate. Part of what makes 92 BPM seem more viable as the primary beat thus relates to what tempos are most ideal or lie near the center of our perceptual window. Research in music cognition, for instance, has shown that ideal tempo lies somewhere within the range of 100–125 BPM.[11] When the rate of kick and snare alternations becomes too slow, therefore, our perception of the primary beat may flip into a higher rhythmic octave to more easily entrain to a steady pulse.

One question the reader might raise here is how to determine whether a song is a “half-time feel” (the snare on beat 3) or simply a slow “normal-time feel” (the snare on beats 2 and 4). My 2016 article addresses some possible factors, such as harmonic rhythm and form, but it is impossible to offer a set of definitive guidelines. Nonetheless, I find that the disbursement of harmonic and melodic content in pop/rock songs tends to occur at a moderate rate; the drum pattern thus often creates the sense (or illusion) of slower or faster tempos, while the melody and lyrics hew to a more moderate pacing. In other words, the tempo implied by the drums might be twice or half that of the tempo implied by the harmonic and melodic content if assessed in isolation from the drums. Some examples will be clearer than others, of course. “Butterfly” by Mariah Carey (1997) and “Get Up on a Room” by R. Kelly (1998), for instance, are fairly unambiguous examples of a half-time 4/4 meter, both at a tempo of 108 BPM. “Radioactive” by Imagine Dragons (2012) is somewhat less clear, although I still hear it as a half-time 4/4 at the rate of 136 BPM. In all three of these cases, one factor that influences my choice of meter is my perception and conception of measure lengths. In “Radioactive,” for example, the harmonic rhythm operates primarily at the rate of one chord per kick-snare alternation. These chords organize into groups of four, with the minor tonic starting each four-chord group. For me, this harmonic grouping delineates the four-bar phrase, which is further reinforced by the melodic structure. It would be possible, I admit, to maintain the same measure lengths and posit a 2/4 or 2/2 meter instead of a half-time 4/4. The difference is simply where one hears the primary beat: Are there only two beats per bar (i.e., a 2/4 or 2/2 meter), each of which aligns with a kick or snare hit, or are there four beats per bar (i.e., a half-time 4/4 meter), with the beats occurring at twice the rate of the kick and snare? I leave such distinctions to the individual analyst, although my own preference is to choose the half-time hearing instead of a time signature change. One central reason is that many songs go back and forth between different drum feels, and thus it seems preferable to maintain a consistent time signature while altering only the drum feel. Along these lines, consider the song “I Wish You Would” by Taylor Swift (2014), which alternates between a normal-time feel in the verse (e.g., 0:08–0:24) and a half-time feel in the chorus (e.g., 0:24–0:36).

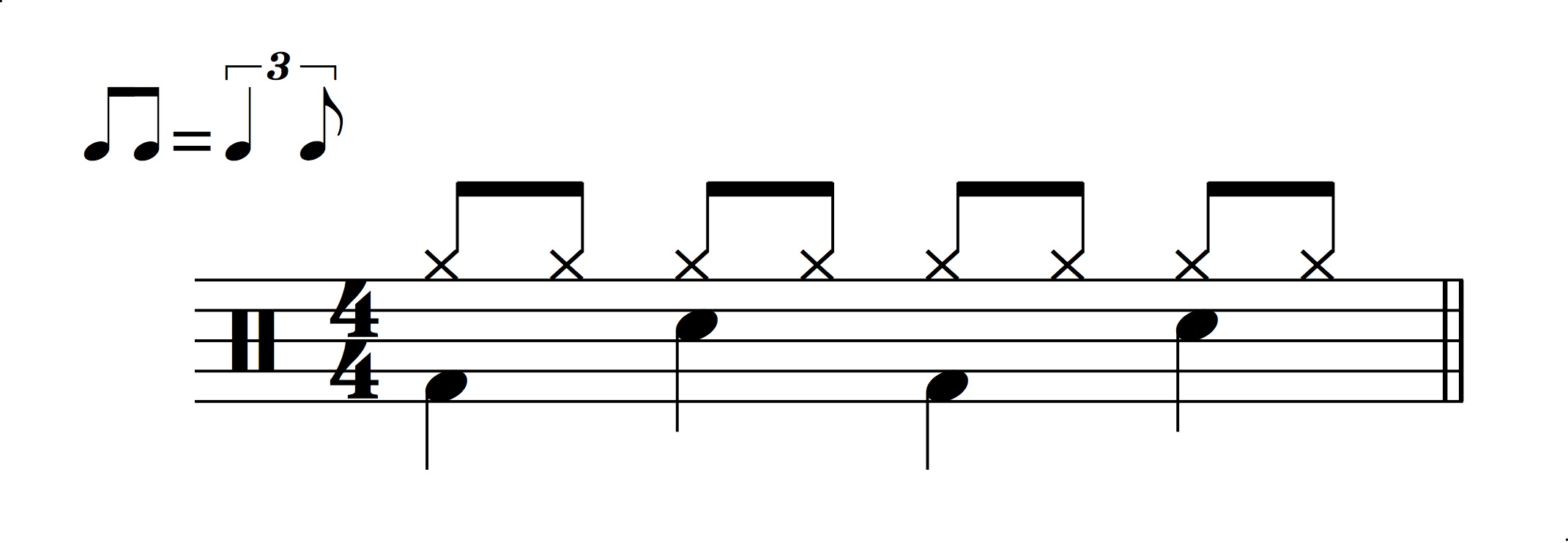
A different though related situation can be found in the song “The Devil Went down to Georgia” by the Charlie Daniels Band (1979). Again, I urge the reader to listen to this song now, and while doing so, consider where the primary beat lies and how the drum pattern interacts with this beat. As I hear it, this song has a main tempo of around 136 BPM. The drums at the opening of the song are thus playing a “double-time feel,” as shown in Figure 4. Like a half-time feel, the kick and snare in a double-time feel alternate at a different metric level than the primary beat—in this case, at a rate twice that of a normal drum beat. As before, the perception of ideal or comfortable tempos affects our hearing of this song as a double-time feel. Specifically, the rate of 272 BPM that would be required to hear the opening of “The Devil Went down to Georgia” as having a standard drum beat is simply too fast to sustain for any extended period of time; most listeners will undoubtedly choose to tap their feet instead at the slower rate of 136 BPM. The drums do eventually revert to a normal-time feel at around 1:26 in the studio recording (when the devil plays his solo with the “band of demons”). Again, the usual practice among popular musicians in such cases is to posit a drum feel change rather than a time signature change.[12]

I will pause the discussion of half-time and double-time feels here. Some of the most interesting metric frameworks arise when these drum feels interact with time signatures other than 4/4 as well as different types of swing rhythms. I will thus shift to a discussion of swing and shuffle rhythms in pop/rock music, after which I will circle back to examples of these drum feels in other metric contexts.

Swing and Shuffle Rhythms Versus Compound Meter

As mentioned previously, the meter of most pop/rock songs can be (and usually is) categorized as 4/4—that is, a simple meter wherein the beat is divided (at least as implied by the time signature) into two equal parts. But in many songs that are notated in 4/4, the beat divisions heard in the sounding music are not, in fact, equally spaced. Instead, the divisions are unequal, such that the subdivision that occurs on the beat is longer than the subdivision that occurs off the beat. For example, consider “I Fall to Pieces” by Patsy Cline (1961). At a moderate tempo of 112 BPM in a 4/4 time signature, this song evinces what pop/rock musicians typically refer to as a “shuffle” rhythm.[13] In the prototypical shuffle rhythm, the divisions of the beat articulate a 2:1 timing relationship, which pop/rock musicians typically represent and conceptualize in one of two ways—namely, either as a notated quarter-eighth triplet within a 4/4 meter or as notated straight eighth notes that are understood to be swung in their performance as quarter-eighth triplets. For the sake of illustration, these two conceptual approaches are represented by the hi-hat part in the drum notation of Figures 5 and 6, respectively.[14] Note that this drum notation is provided here for illustration purposes only; typically, all members of the ensemble will participate in the shuffle rhythm if it is present in the music.

I take Figures 5 and 6 to be essentially equivalent representations of the same rhythm, differentiated only by notational preference. It would also be possible to notate the shuffle rhythm as shown in Figure 7—that is, using a 12/8 time signature such that the quarter-eighth subdivisions align with the normative division scheme of a compound meter. Yet the notation in Figure 7 is generally deprecated among pop/rock musicians, even among those with formal academic training in music.[15] In other words, pop/rock musicians tend to prefer thinking about shuffle rhythms in a simple quadruple meter rather than in a compound quadruple meter. The difference between Figure 7 and the other two representations of the shuffle rhythm in Figures 5 and 6 might seem trivial, but I believe the preference for a 4/4 time signature is an important and meaningful shift among pop/rock musicians in terms of thinking about rhythm and meter.

One central reason that 4/4 is preferred to 12/8 might relate to the exact timing of the beat divisions. Specifically, a compound meter such as 12/8 implies three equal divisions of the beat. In contrast, shuffle and swing rhythms in pop/rock can be considered two unequal divisions of the beat.[16] That is to say, the triplets (or implied triplets) in Figures 5 and 6 serve only as approximations of the actual sounding rhythms. Although the conventional assumption for swung eighth notes is that they reflect an underlying triple pulse and should be performed in a 2:1 ratio, recent studies of jazz performances have shown that the ratio between swung eighth notes in practice will vary anywhere from 1:1 (i.e., straight eighth notes) to 3.5:1 (i.e., a doubly dotted eighth note followed by a thirty-second note).[17] Butterfield elegantly sums up the situation when he writes, “Swing is not a specifiable quantity . . . nor is it a quality that is precisely quantifiable” (2011, 24).[18] We should surmise, therefore, that swing and shuffle rhythms in pop/rock music might not correspond exactly to the 2:1 configuration either.

In reality, when listening to shuffle and swing rhythms in pop/rock music, it is usually difficult to determine solely by ear the exact timing of the subdivisions. In “I Fall to Pieces,” for example, I perceive the shuffle rhythm to be very close to a 2:1 ratio. But is it exactly 2:1, or is there a subtle yet pervasive timing discrepancy that is responsible for the overall metric quality of the song? Without loading the song into a digital audio workstation, I have no obvious way to know. Even if I looked at the song more closely using a computer, a certain amount of estimation would be required to identify the precise location of each note. I might take the clear triplet rhythm in the opening guitar part of “I Fall to Pieces” as evidence for a consistent 2:1 ratio, but a fleeting moment of evenly spaced triple subdivisions does not necessarily mean that a 2:1 ratio is maintained throughout the song. For instance, the swing ratio of the ensemble in “Girl They Won’t Believe It” by Joss Stone (2007) is obviously not in a 2:1 ratio; instead, the swing occurs at something like a 3.5:1 relationship, according to my own measurements. Despite this pervasive “hard swing,” the drum fill prior to the first chorus (around 0:49) switches to what sounds like evenly spaced triplets, if only briefly. It is possible, therefore, that swing ratios change during different sections of the song—or possibly, different instruments might swing at different ratios.[19] In PJ Harvey’s “The Words That Maketh Murder” (2011), for example, Azevedo et al. (2015) find that the autoharp is swung while the drums articulate a straighter eighth-note pattern.

Because there is no notated score to a pop/rock song, it is thus usually unclear how the beat precisely divides in any given recording. Accordingly, I posit that there should be three ways to classify meter on the basis of the beat division: (1) as simple, where the beat is divided into two equal parts; (2) as compound, where the beat is divided into three equal parts; or (3) as swung, where the beat is divided into two unequal parts. When a quadruple meter is swung, it seems preferable to conceive of it in a 4/4 time signature rather than a 12/8, if only because the 12/8 time signature implies a strict division of the beat into three equal parts. To be clear, I do not mean to posit that there are never cases in which a song seems to warrant a compound quadruple meter. “Call Me” by Blondie (1980), “Everybody Wants to Rule the World” by Tears for Fears (1985), “When the Going Gets Tough, the Tough Get Going” by Billy Ocean (1985), and “I’m Your Baby Tonight” by Whitney Houston (1990) are good examples of songs in which the three-part division of the beat is consistent and clear throughout the song. But songs like this are much less frequently encountered in pop/rock music than those with shuffle and swing rhythms, perhaps because the binary up-down strumming pattern typically used on a guitar creates an inherent preference for duple divisions over triple divisions.

Although incontestable compound quadruple meters (12/8) might be somewhat rare in pop/rock music, compound duple meters (e.g., 6/8) are more common. One reason for this difference might derive from the way in which our perception of the primary beat is influenced by our limited window for comfortable tempos. According to conventional wisdom, for instance, it is a fundamental misunderstanding of 6/8 to hear the eighth note as the beat.[20] This explanation does not, however, coincide with the way many pop/rock musicians, including drummers, explain their own hearings of 6/8. For example, Jim Riley—the bandleader and drummer for Rascal Flatts as well as a graduate of the University of North Texas with a degree in music education—writes that 6/8 has “six beats per measure with the eighth-note pulse representing each beat” (2010, 28). Similar statements can be found in Matthews (1984, 45) and Williams (2012, 12). We could say, of course, that these professional musicians (all of whom studied music in college) are misconstruing the nature of 6/8—that they either are ignorant of or have forgotten basic tenets of their musical education. Yet these musicians are describing where they perceive the beat, and it would be somewhat unsympathetic to disregard how they report their own perception.

As a point of reference, consider the song “Nothing Else Matters” by Metallica (1991), which I consider to be a classic example of 6/8.[21] The drum pattern in this song illustrates the standard pattern of kick and snare in pop/rock music for a 6/8 meter, as shown in Figure 8. If we take the kick and snare to imply the primary beat level of the song (i.e., the traditional approach), then we would say that “Nothing Else Matters” has a tempo of around 48 BPM—near the lower limit for beat perception.[22] The reader should notice, however, that the standard 6/8 beat is more similar to a half-time 4/4 feel than a standard 4/4 feel, in that each bar has only one kick and snare instance. In other words, 6/8—like a half-time 4/4—potentially presents the listener with a conflict between two metrical levels. For example, the rate of the eighth note in this song (as articulated by the hi-hat part) is about 144 BPM—much closer to estimates of ideal tempo than the 48 BPM rate articulated by the kick and snare. I do not mean to imply here that we should necessarily abandon hearing the dotted quarter note as the beat. Rather, the dotted quarter is one level of beat, and there is a complementary level of beat that can be felt on the eighth-note level.

The ability to hear the eighth note as the beat in a 6/8 meter contrasts strongly with the typical situation in a 12/8 meter. Compare “Nothing Else Matters,” for example, to the Blondie song “Call Me” mentioned previously. In both songs, the eighth-note pulse is consistently articulated in the music. But the speed of the eighth note in “Call Me” is tremendously fast—somewhere around 426 BPM if we were to take the eighth note as the beat—and thus far beyond the limits for beat perception.[23] In “Call Me,” therefore, it is clear that the dotted quarter is the beat (assuming a meter of 12/8), which results in a tempo of around 142 BPM. Note that this tempo is almost exactly the same tempo as the eighth note in “Nothing Else Matters” (around 144 BPM). The 12/8 in “Call Me” and the 6/8 in “Nothing Else Matters” are thus very different types of compound meters. In “Call Me,” we can hear only the dotted quarter as the beat, whereas in “Nothing Else Matters”—even though it is possible to hear the dotted quarter as the beat—our perceptual window for tempo encourages us to hear the eighth note as the beat.

For popular music, the concept of compound meter is thus well suited to describe triply divided duple meters, since the eighth note can often be heard as the beat itself; in contrast, the concept of compound meter is somewhat unnecessary for triply divided quadruple meters, since the fast subdivision can be conceptualized instead as a pervasive triplet division of a quarter note beat. Wyatt, Schroeder, and Elliott summarize this sentiment in their ear training manual for contemporary musicians, writing that “in popular music, compound meter is generally used only at slower tempos; when the tempo picks up, the triplet feeling is better defined as shuffle or swing” (2005, 77). Part of the issue is that as the tempo of a compound meter increases, the middle note of the triple division typically gets dropped; as a result, there arises an inherent ambiguity with regard to the precise division of the beat, such that it is preferable to think of the meter as 4/4 with swing instead of a strict compound quadruple.

The Interaction of Drum Feels and Swing Rhythms in 4/4

In the previous sections, I discussed two additional factors—drum feels and swing rhythms—that I take to be important aspects of meter in pop/rock music beyond the time signature itself. Considered in isolation, each of these factors might not seem to signal any significant shortcoming with traditional time signatures. It is when these two factors combine, however, that time signatures alone more obviously fall short of modeling the metric hierarchy of a song. In the current section, I consider the interaction of drum feels and swing rhythms in 4/4; in the next section, I look at the interaction of these two factors in other time signatures.

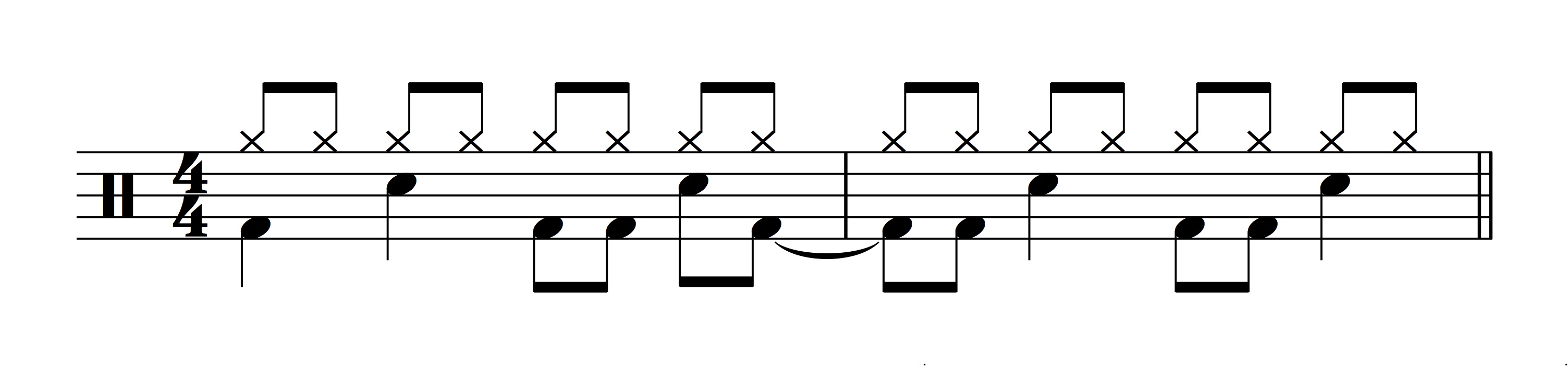
To my ears, one of the most aurally compelling metric organizations in pop/rock music arises from the combination of a half-time 4/4 feel with swung eighth notes, as shown in Figure 9—a groove that is typically referred to as the “half-time shuffle.” The half-time shuffle has also been called the “Purdie shuffle” in honor of drummer Bernard Purdie, who plays this beat on the song “Home at Last” by Steely Dan (1977).[24] “Home at Last” is not the first instance of the half-time shuffle in history; earlier instances include the 1968 live recording of “Please Return Your Love to Me” by the Temptations and the 1972 song “Loose Booty” by Funkadelic (Zoro 2013, 12). But “Home at Last” has become an iconic instance of the half-time shuffle, perhaps because of how clearly the drum part implies a half-time feel against (or combined with) the shuffle subdivision of the primary beat. Specifically, the 126 BPM rate for the main beat lies very near ideal tempo, and so the listener is encouraged to hear the primary beat on a different level of the metric hierarchy than the kick and snare alternations.

It is important to note that the metric organization of a half-time shuffle is impossible to fully convey using only traditional time signatures. To be sure, we could notate Figure 9 as in 12/8, but doing so would not reflect the tension between the perceived primary beat and the kick and snare pattern. Alternately, we could notate Figure 9 in a duple meter so as to coincide with the kick and snare, but then there would be no way to reflect the persistent triple-based divisions of the hi-hat rhythm in the time signature itself. (Compound meters only allow for triple divisions at the level immediately below the beat.) One might argue that traditional time signatures are not meant to indicate all these aspects of rhythm and meter; I would counter that this is the exact limitation of using traditional time signatures alone with popular music. To fully capture the complete rhythm and metric organization of a song like “Home at Last” requires language that goes beyond the time signature itself.

Because a half-time shuffle relies on hearing the kick and snare as alternating at half the rate of the primary beat, most classic half-time shuffles employ speeds near the center of our perceptual window for tempo. In “Fool in the Rain” by Led Zeppelin (1979), for instance, drummer John Bonham clocks in at around 130 BPM; similarly, the half-time shuffle in “Nothing Compares 2 U” by Sinéad O’Connor (1990) has a primary beat rate of about 120 BPM. In the song “Rosanna” by Toto (1982), drummer Jeff Porcaro speeds the half-time shuffle up to around 172 BPM.[25] At this tempo, the listener might be tempted to hear the primary beat at half this rate—that is, 86 BPM—thus aligning with the kick and snare. Indeed, this faster tempo starts to push the limit of our perception of this particular groove as a half-time feel. That said, the contrasting section in the song, which first occurs at around 0:45, switches back to a normal-time feel (thus providing more evidence for hearing the fast tempo); documentary evidence from Porcaro also shows that he conceives of the tempo at the faster rate of 172 BPM.[26]

As the half-time shuffle continues to increase in tempo, it becomes easier and easier to hear the kick and the snare as aligning with the primary beat. Consider in this regard the song “Been Caught Stealing” by Jane’s Addiction (1990). I encourage the reader to listen to this song now, and while doing so, think about how to best classify its metric organization. To my ears, the song sounds like a normal-time 4/4 at a moderate tempo of around 104 BPM, and I believe this hearing would be shared by most listeners. But what might not be obvious is the pervasive swing at the sixteenth-note level (assuming a 4/4 meter at 104 BPM). Swing at the sixteenth-note level in a normal-time 4/4 can be seen, arguably, as the result of the half-time shuffle speeding up even faster than the “Rosanna” example. For instance, it is not impossible to hear “Been Caught Stealing” as a half-time shuffle at a tempo of 208 BPM. But the melodic phrase structure and the harmonic rhythm, combined with our preference to hear the main pulse at a more moderate rate, encourage us to hear the song as a normal-time feel at the tempo of 104 BPM, such that the sixteenth notes rather than the eighth notes are “shuffled.”

Swing at the sixteenth-note level in a normal-time 4/4 can be a subtle effect, perhaps because traditional time signatures tend to encourage us to think primarily in terms of the beat level and the first level of division below this beat. In addition, the hi-hat might only articulate the straight eighth-note pulse, leaving us to infer the swung sixteenth notes from the rhythmic motives in other instruments. But once a listener becomes used to hearing half-time shuffles at moderate tempos, sixteenth-note swing should aurally evoke a similar metric hierarchy—that is, that swing is occurring on a pulse layer two levels down from the kick and snare alternations. Other examples of sixteenth-note swing in normal-time 4/4 include “Poison” by Bell Biv DeVoe (1990), “Someday” by Mariah Carey (1990), and “Love on Top” by Beyoncé (2011). The swung sixteenth in a normal-time 4/4 is particularly characteristic of the “new jack swing” movement to come out of New York City in the late 1980s and early ’90s, catalyzed perhaps in large part by the ability to program a swung sixteenth note into drum sequencers of the time.

Swung sixteenth notes can also be combined with a double-time 4/4 drum feel to create yet another possible metric organization. A double-time 4/4 with swung sixteenths could also be heard as a sped-up version of a normal-time 4/4 shuffle. For example, consider the song “Redneck Woman” by Gretchen Wilson (2004). It is possible to hear the tempo of this song as around 184 BPM, with the kick and snare articulating the main beat of the song. With this hearing, the eighth-note shuffle rhythm in the rhythm guitar and drum fills should be clear. As I conceptualize the song, though, the primary beat level is around 92 BPM, such that the kick and snare are alternating at twice the rate of my foot tapping. Hearing the song at 92 BPM makes much more sense in terms of form as well, since the verse and chorus sections become twelve-bar modules that closely mirror the harmonic structure of a twelve-bar blues. The eighth-note shuffle rhythm at the 184 BPM tempo, therefore, turns into swung sixteenths at 92 BPM in this double-time hearing. Other songs, such as “Up from Below” by Edward Sharpe and the Magnetic Zeros (2009), more clearly evince this swung sixteenth, double-time 4/4 framework due to the increased tempo that further encourages us to hear the kick and snare at a metric level above the primary beat.

The Interaction of Drum Feels and Swing in 6/8 and 3/4

In this last main section of my chapter, I consider how various types of swing rhythms and drum feels interact with time signatures other than 4/4. Specifically, I focus here on 6/8 and 3/4, in part because it is sometimes difficult to determine which of these two time signatures best describes the metric hierarchy of a song. This ambiguity, as we will see, derives from the way in which a nonnormative drum feel can blur the boundary between 6/8 and 3/4.

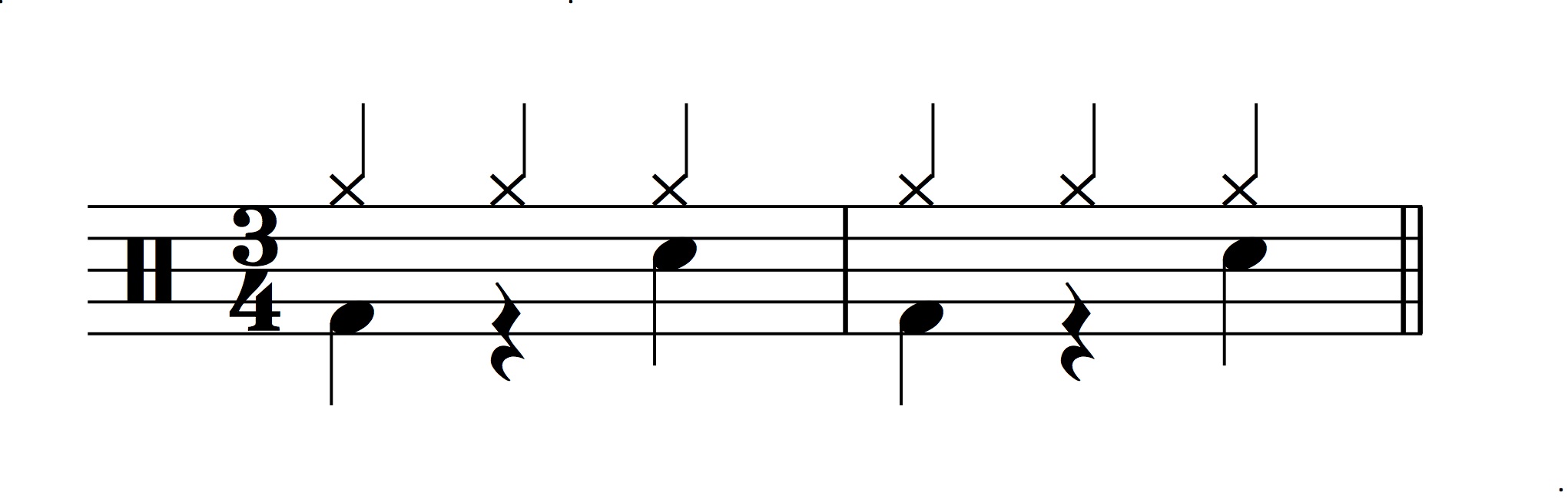
I will begin with a discussion of 3/4, since I have not yet described its normal drum feel. As shown in Figure 10, the standard drum pattern for a song in 3/4 involves a kick on beat 1 and a snare on beat 3; beat 2 is shown as a rest, although drummers will often play either a kick or a snare on this beat as well. The song “No Other One” by Weezer (1996) is one of the clearest examples of 3/4 in pop/rock music, due primarily to the harmonic rhythm of the song and the moderate tempo of 100 BPM.[27] Instances of 3/4 in pop/rock music are relatively rare, at least in comparison to 4/4 and 6/8, perhaps in part because of the unequal (or nonbinary) alternation of kick and snare in a simple triple meter. That is to say, in both 4/4 and 6/8, the kick and snare are consistently separated by equal intervals of time, whereas in a normal-time 3/4, the possibility to evenly space the kick and snare is thwarted (assuming the snare lands on a beat), thereby creating a somewhat lopsided sensation.

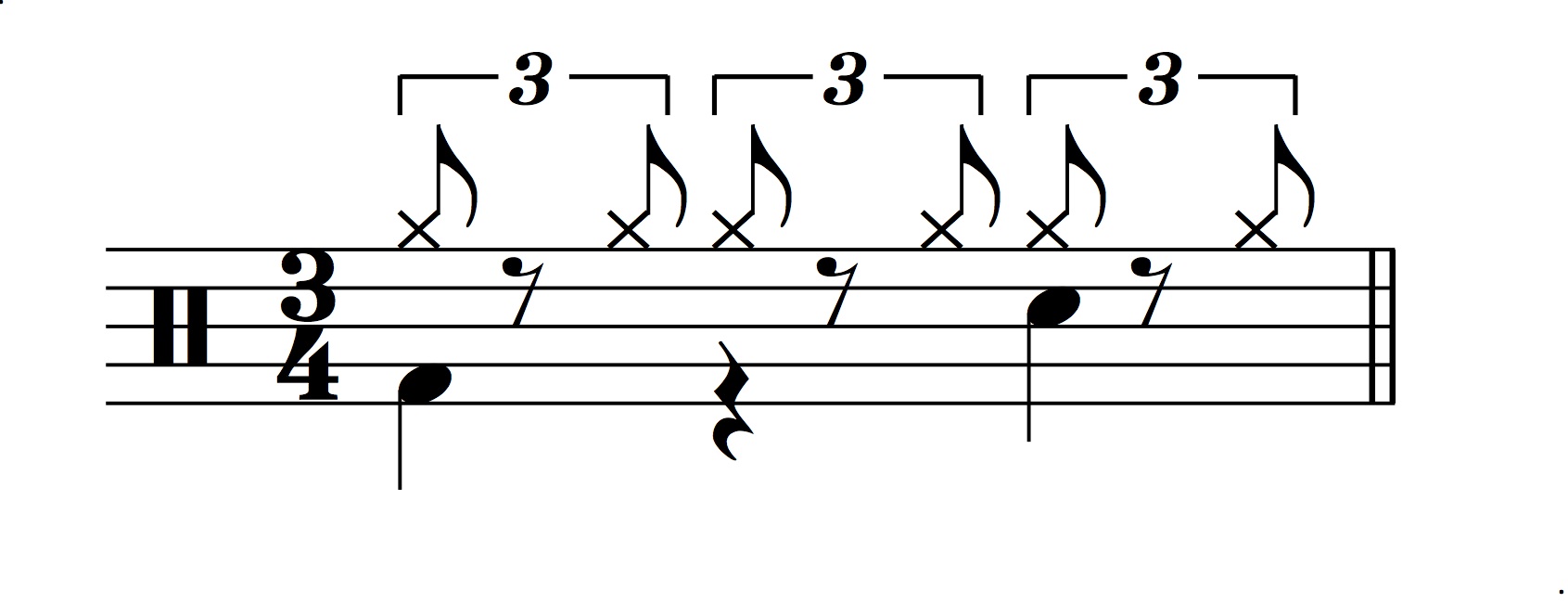
In my experience, pop/rock songs in 3/4 more often than not include swing on the eighth-note level, as approximated in Figure 11. This metric framework can be found in songs such as “I Never Love a Man (the Way I Love You)” by Aretha Franklin (1967) and “(Who Says) You Can’t Have It All” by Alan Jackson (1992), both of which have a moderate tempo of about 90 BPM. It would be possible, of course, to notate the rhythm in Figure 11 in a time signature of 9/8. But as discussed previously, it is difficult to tell whether the timing of the hi-hat (or other percussive part, such as the rhythm guitar) is precisely in a 2:1 ratio, so 3/4 seems preferable as the primary time signature.[28]

Swing rhythms in a 6/8 time signature cannot occur at the eighth-note level (the meter would cease to be compound), but swing often occurs in 6/8 at the sixteenth-note level. Like sixteenth-note swing in 4/4, sixteenth-note swing in 6/8 can be subtle. Typically, though, the hi-hat pattern or rhythm guitar gives away the unequal lengths of the sixteenth-note subdivisions. For illustration purposes, Figure 12 depicts the triplet-based approximation of a normal 6/8 drum pattern with sixteenth-note swing, wherein the swung sixteenth note is articulated by the hi-hat on the middle eighth note of each dotted-quarter beat. The song “Trouble” by Ray LaMontagne (2004) is a good example of this metric organization, most obviously at the beginning of the song. Note that while the drummer for “Trouble” often retreats to a straight eighth-note pattern on the hi-hat, the rhythm guitar continues to articulate swung sixteenth notes, which give the song an extra lilting quality beyond the usual lilt of a 6/8. Other good examples of 6/8 meters with swung sixteenth notes include the cover of “With a Little Help from My Friends” by Joe Cocker (1969), “Breaking the Girl” by the Red Hot Chili Peppers (1991), and “Paperwork” by T.I. (2014). For those readers (or their students) that might have trouble hearing sixteenth-note swing in 6/8, I recommend trying to hear the eighth note as the beat, since it is usually easiest to hear swing at the metric level just below the main pulse.

Different types of drum feels can also occur in 3/4 and 6/8, with or without the addition of swing rhythms. As in 4/4, these drum feels result in a shifting of the kick and snare alternations up or down a level in the metric hierarchy as compared to their normal-time versions. Variations in drum feels with time signatures of 3/4 or 6/8 are admittedly not common metric organizations due to the relative rarity of 3/4 and 6/8 time signatures overall (at least in comparison to 4/4). But this rarity only makes the instances found in real songs that much more fascinating. Consider, for example, the song “Synchronicity” by the Police (1983). Most if not all transcriptions of this song cast it in a time signature of 6/4, which is problematic for at least two reasons.[29] First, a 6/4 time signature would imply—at least in its usual understanding—a compound duple meter (with the dotted half note getting the beat), which obviously does not match the metric organization of the song.[30] Second, if we are to understand the 6/4 time signature as an extended bar of 4/4, then this implies a tempo of around 200 BPM, which although possible, lies at about twice the value for ideal tempo. As I hear it, “Synchronicity” is a double-time 3/4 at the more moderate tempo of 100 BPM, as modeled in Figure 13.[31] Some evidence for this hearing is provided by the lead singer, Sting, who counts off the song in concert as “one, two, three” at a moderate tempo.[32] Note that a double-time 3/4 hearing maintains the same measure lengths as the 6/4 hearing, so the phrase structure of the song is unaffected. But rather than employing (somewhat awkwardly) a nonstandard time signature such as 6/4, we can understand the metric organization of the song as a combination of standard metric parameters.

In addition to a double-time feel, 3/4 can also be organized as a half-time feel, as shown in Figure 14. In a half-time 3/4, the kick occurs on the downbeat of every other measure instead of every measure—that is, half as often. The song “Dig a Pony” by the Beatles (1970)—which includes a “one, two, three” count off at the beginning of the studio recording and has been analyzed as occurring in triple meter by various authors, including Fujita et al. (1989, 194), Pollack (1993), and Everett (2009, 305)—provides a good example of a half-time 3/4. Note that “Dig a Pony” also includes swing on the eighth notes. In fact, all instances of half-time drum feels in 3/4 that I have encountered include swung eighth notes, including “Still Crazy after All These Years” by Paul Simon (1975), “If It’s Over” by Mariah Carey (1991), and “Sometimes I Cry” by Chris Stapleton (2015).

It is worth noting that a half-time 3/4 could be heard as a normal-time 6/8, with the quarter note in 3/4 equaling the eighth note in 6/8. Similarly, a double-time 6/8, as shown in Figure 15, might alternatively be heard as a normal-time 3/4, with the eighth note in 6/8 equaling the quarter note in 3/4. The difference can sometimes be ambiguous, especially if one is acclimated to hearing the eighth note as the pulse in 6/8. In this regard, consider the song “Take It to the Limit” by the Eagles (1975). Based on the drum pattern at the beginning of the song, we might initially posit a normal-time 6/8 with swung sixteenths at a tempo of 30 BPM for the dotted quarter note. With this hearing, the change in the drum pattern at 1:12 (just prior to the chorus) would be considered a double-time 6/8 with swung sixteenths. Alternatively, we could posit that the opening of the song is a half-time 3/4 with swung eighths at a tempo of 90 BPM, after which the drums shift to a normal-time feel at 1:12.[33] Ultimately, our choice of which reading is best affects the measure lengths we posit for this song and thus its overall form (the 6/8 reading will have half as many measures as the 3/4 reading). As the reader might have guessed, I believe the 3/4 reading is highly preferable in this case, since it creates measure lengths of exactly two seconds. (The tempo of 30 BPM also lies at the extreme limit of perceptible beat rates.) If the pacing of the song were different, however, we might make a different choice.

As a final musical example, take the song “Lorelai” by the Fleet Foxes (2011). Based on the opening drum pattern, we might assess the song to be in 3/4 at a tempo of 145 BPM, which generates measure lengths of around 1.24 seconds. This metric interpretation, however, creates a serious misrepresentation of the song’s form. Reading the song in 3/4, the first large vocal passage—spanning from about 0:34 to 1:14—would last thirty-two bars, and due to the melodic and harmonic structure of these bars, we would say that it is a thirty-two-bar AABA form. Yet this vocal passage includes none of the structural weight that we expect of a thirty-two-bar AABA; the final A is more of an afterthought than a return of a full-fledged section, and there is simply not enough musical content to warrant the thirty-two-bar AABA label. Instead, it seems more appropriate to consider this vocal passage as a sixteen-bar SRDC.[34] To do so, we must posit that “Lorelai” evinces a double-time 6/8 feel (with the eighth note equal to 145 BPM), with measure lengths twice as long as the normal-time 3/4. With this reading, the opening line (from “So guess I got old” to “sidewalk”) constitutes a four-bar phrase, which seems like a more appropriate length for its melodic and lyric content.

Conclusion

The preceding paragraphs have moved through a number of musical examples, as well as a number of different metric organizations, and thus the reader might benefit from a top-down view of the entire scheme that I have described. In essence, my system classifies meter in pop/rock music using three basic categories, each of which has three standard options: (1) the time signature, which is usually 6/8, 3/4, or 4/4; (2) the drum feel, which can be normal-time, half-time, or double-time; and (3) the extent of swing, which can be none (straight), swing on the eighth notes, or swing on the sixteenths. Classifying the meter of a song requires choosing one (and only one) option from each of these three categories. A given song, for example, might be a normal-time 6/8 with swung sixteenths; another might be a half-time 3/4 with swung eighths. This classification system is relatively uncomplicated—only three categories, each of which has three standard options—but it allows us to describe the metric organization of a song in a relatively sophisticated way. Specifically, there are twenty-two viable combinations using this method.[35] This variety allows for a great degree of analytic flexibility, especially compared to the six standard time signatures used in traditional practice (i.e., 2/4, 3/4, 4/4, 6/8, 9/8, and 12/8).

The reader will probably have noticed that the system described above offers only three standard options for the time signature: 6/8, 3/4, and 4/4. I freely admit that some songs are best represented by other time signatures, such as 12/8 or 2/4. Similarly, I remind the reader that I have avoided any discussion of asymmetrical, complex, or odd meters, and I did not delve into changing meters or polymeters. My goal here has not been to provide an exhaustive discussion of all possible metric organizations found in pop/rock music; rather, I have intended to provide a blueprint for expanding the foundation of meter classification more generally. With this goal in mind, the time signatures of 6/8, 3/4, and 4/4 are able to account for the vast majority of songs in pop/rock music, because these three time signatures account for the most basic combinations of beats and divisions commonly found in pop/rock: two beats with two divisions (4/4), two beats with three divisions (6/8), and three beats with two divisions (3/4). For whatever reason, the remaining combination—three beats with three divisions (9/8)—is so rarely encountered in pop/rock music that I do not consider it to be a standard choice (although I do not deny that it is possible).[36]

Given the variety of possible metric organizations described previously, the reader might expect a long appendix of additional musical examples. Due to considerations of space, I have opted to post this appendix online at http://www.midside.com/skills/rhythm/_meter_id/. Having the appendix online allows me to provide audio files for each example as well as to continue adding examples as they are found. That said, I believe the reader should now be equipped to hear instances of these various metric organizations in their day-to-day listening of pop/rock music, and so I hope the reader begins to find new and interesting examples on their own.

In summary, I believe the approach I have described herein represents a new way of hearing and thinking about meter in pop/rock music. There are many ramifications of adopting this approach that remain open questions. For example, should we teach students how to perform rhythms with swing, both at the eighth-note and the sixteenth-note level? As rhythm reading is conventionally taught in an aural skills curriculum, rhythms are always performed “straight,” with no swing. Similarly, should students—especially those in pop music or music industry curricula—be required to re-create different types of drum feels (akin to conducting) while performing rhythms? Ultimately, a large gap still remains between the skill sets that are developed in the traditional music classroom and those that might be relevant to musicians working with pop/rock materials. Hopefully, this chapter presents a useful conceptual framework to help bridge that gap.

Notes

1. For a few different perspectives on the validity of functional harmony in rock music, see Moore (1992), Stephenson (2002), Everett (2004), and de Clercq and Temperley (2011).

2. Similar to the approach in Covach (2009), I adopt a broad definition of “pop/rock” music in this chapter, using the term to encompass most Anglo-American popular commercial music styles aimed at a youth audience, including but not limited to rock, R&B, country, hip-hop/rap, blues, pop, and folk.

3. I have closely paraphrased here the definition of meter categorization as presented in the Grove Dictionary of Music (London 2016).

4. In my 2016 article, I look at three corpora of popular music—Covach (2009), Burgoyne et al. (2011), and Temperley and de Clercq (2013)—and find that 4/4 is by far the most common meter chosen by the analysts.

5. Throughout this chapter, I will use standard drum notation, in which the kick drum is notated on the bottom space of a five-line staff, the snare on the second space from the top, and the hi-hat on the space above the top line.

6. Temperley (1999) argues that anticipations to the beat can be normalized (or “de-syncopated”) so as to conceptually belong to the beat that follows. Thus the kick that occurs one eighth note prior to the downbeat of measure two in Figure 2 can be considered to be a displaced version of the downbeat.

7. For examples of how the standard rock beat is taken as a model for measure lengths and time signatures, see the discussions in Moore (2001, 42) and Stephenson (2002, 2).

8. Throughout this chapter, I will cite songs to exemplify and illustrate different meter types. I eschew transcriptions of these songs, primarily due to copyright concerns although also because a transcription can never fully capture the sound and feel of the recording. Accordingly, it is best if the reader listens to each of these songs, which is easy to do nowadays through YouTube, Spotify, or some other online source. I will always specify the tempo (in BPM), time signature, and drum feel of each song so that the reader can hear these examples in the same manner as I do.

9. This terminology can be found in a variety of publications authored by professional drummers, including Morgenstein and Mattingly (1997), Mattingly (2006), Riley (2010), and Berry and Gianni (2012).

10. The reason for foot-tapping or head-bobbing at this particular rate relates to issues of embodiment, which are described in Iyer (2002).

11. Research reported in London (2012)—such as work by Fraisse (1982), Parncutt (1994), and Semjen, Vorberg, and Schulze (1998)—suggests that ideal tempo lies around 100 BPM. More recent work by Moelants (2002), Moelants and McKinney (2004), and Levy (2011) suggests that ideal tempo lies closer to 120 or 125 BPM. As reported in my 2016 article, average tempo for pop/rock songs in 4/4 is somewhere close to 120 BPM.

12. See, for example, the chart to “Feeling’s Gone” in Riley (2010, 32).

13. Most of the transcriptions of this song, found with a Google image search of the words “sheet music i fall to pieces,” set the song in a meter of 4/4 (accessed December 2, 2016).

14. Figure 5 models the standard notational approach to shuffle rhythms used in the pop/rock drum literature, as found in Morgenstein and Mattingly (1997, 61), Potter (2001, 7), Mattingly (2006, 39), Zoro (2007, 37), Berry and Gianni (2012, 32), Zoro (2013, 22), and Riley (2015, 33). Figure 6 models the standard notational approach to shuffle rhythms used in vocal/guitar/piano songbooks, such as Randall and Peterson (1997, 23).

15. Jim Riley has a degree in music from the University of North Texas, for example, yet in his 2015 book, he uses the notation shown in Figure 5 for swing rhythms.

16. I take the terms “swing” and “shuffle” to be essentially synonymous for the purposes of this chapter, although “shuffle” seems to usually imply a type of swing rhythm that hews closely to the 2:1 ratio (Riley 2015).

17. One important metastudy on swing ratios in jazz can be found in Butterfield (2011). This article surveys a variety of other research on jazz microtiming, including work by Friberg and Sundström (2002) and Benadon (2006), who find that 2:1 swing ratios are more often the exception than the norm in performance practice.

18. It may also be worth emphasizing that “swing” as a term encompasses much more than simply a timing relationship between beat divisions, which is why I refer to these as swing “rhythms,” not simply as “swing.”

19. For jazz performances, Butterfield (2011) finds that soloists typically have swing ratios less than 2:1, while rhythm section players typically have swing ratios greater than 2:1.

20. See, for example, the length taken in Kostka, Payne, and Almén (2013, 30–31) to make clear to the student that 6/8 does not have six beats in the measure.

21. Most of the transcriptions of this song, found with a Google image search of the words “sheet music metallica nothing else matters,” set the song in a meter of 6/8 (accessed May 17, 2016).

22. London (2012, 30) puts the floor on realistic rates of beat perception at around 30 BPM.

23. London (2012, 30) puts the ceiling on realistic rates of beat perception at around 240 BPM.

24. This is the provenance of the half-time shuffle as explained in Zoro (2013, 14).

25. Interestingly, Jeff Porcaro was the drummer for Steely Dan prior to Bernard Purdie, even though—by Porcaro’s own admission—he “stole” the beat for “Rosanna” from Bernard Purdie, as documented in “The Rosanna Half Time Shuffle by Jeff Porcaro,” YouTube video, posted by “Mark S.,” August 27, 2011, https://www.youtube.com/watch?v=pwyO1qr0edI.

26. In a Drummerworld video on “Rosanna,” Porcaro’s discussion shows that he clearly conceives of the beat as in 4/4 with triplets and the snare on beat 3 (“Rosanna Half Time Shuffle”).

27. Rosenberg (2011) classifies “No Other One” as in 6/4, but I am not sure if she is hearing the hi-hat as the beat at a tempo of 200 BPM or measure lengths that are twice as long as in my reading. Either way, I think this is an incorrect categorization of the meter for this song.

28. Note that some of the songs Rosenberg (2011, 65) classifies as 3/4—for example, “Manic Depression” by Jimi Hendrix (1967) and “Miss Misery” by Elliot Smith (1997)—have swung eighth notes, yet Rosenberg does not classify these songs (rightly so, I would say) as in 9/8.

29. Specifically, a Google image search of “synchronicity police sheet music” shows all versions to be in 6/4 (accessed May 19, 2016).

30. The idea that 6/4 is typically a notational variant of 6/8 can be found in various music theory textbooks and rhythm readers—for example, Kostka, Payne, Almén (2013, 30) and chapter 15 of Hall (2005).

31. The pattern in Figure 13 could be called a triple-time 3/4 in that the kick and snare are alternating at three times the normal-time rate. But since the kick and snare imply a tempo that is only twice the primary beat and double-time is the standard term used in 4/4, I prefer to refer to this pattern as a double-time 3/4.

32. See the various live videos available on YouTube of their November 3, 1983, concert in Atlanta, Georgia, at the Omni Coliseum. For example, “The Police—‘Synchronicity I’ Live,” YouTube video, posted by “SenseiSlaughter666,” August 30, 2011, http://www.youtube.com/watch?v=FMmCJ6-uu3M.

33. I suppose there exists a third option for those readers that prefer the drum pattern to imply a normal-time feel, such that the song would begin in 6/8 and then switch to 3/4 at 1:12, with the eighth note in the 6/8 being equal to the quarter note in the 3/4. This approach mangles the form of the song, however, in my opinion.

34. SRDC is a term coined by Everett (2009, 140) that refers to a phrase structure of “Statement-Restatement-Departure-Conclusion,” somewhat akin to the classical-era sentence. For a deeper discussion of AABA and SRDC forms, see my 2012 dissertation.

35. Three categories with three standard options give twenty-seven theoretical possibilities (3 × 3 × 3), but there are really only twenty-two possible combinations because 6/8 never has swung eighth notes and never seems to occur as a half-time feel.

36. Everett (2009, 305) confirms that compound triple meter is “virtually nonexistent” in pop/rock music.

References

• Azevedo, Cláudia, Chris Fuller, Juliana Guerrero, Michael Kaler, and Brad Osborn. 2015. “An Ambiguous Murder: Questions of Intertextuality in PJ Harvey’s ‘The Words That Maketh Murder.’” In Song Interpretation in 21st-Century Pop Music, edited by Ralf von Appen, André Doehring, Dietrich Helms, and Allan F. Moore, 175–95. Burlington, VT: Ashgate.
• Benadon, Fernando. 2006. “Slicing the Beat: Jazz Eighth-Notes as Expressive Microrhythm.” Ethnomusicology 50 (1): 73–98.
• Berry, Mick, and Jason Gianni. 2012. The Drummer’s Bible: How to Play Every Drum Style from Afro-Cuban to Zydeco. 2nd ed. Tucson, AZ: See Sharp Press.
• Biamonte, Nicole. 2014. “Formal Functions of Metric Dissonance in Rock Music.” Music Theory Online 20 (2).
• Burgoyne, John, Jonathan Wild, and Ichiro Fujinaga. 2011. “An Expert Ground-Truth Set for Audio Chord Recognition and Music Analysis.” In Proceedings of the 12th International Society for Music Information Retrieval Conference (ISMIR), October 24–28, edited by Anssi Klapuri and Colby Leider, 633–38. Miami: University of Miami.
• Butler, Mark. 2001. “Turning the Beat Around: Reinterpretation, Metrical Dissonance, and Asymmetry in Electronic Dance Music.” Music Theory Online 7 (6).
• Butterfield, Matthew. 2011. “Why Do Jazz Musicians Swing Their Eighth Notes?” Music Theory Spectrum 33 (1): 3–26.
• Covach, John. 2009. What’s That Sound? An Introduction to Rock and Its History. 2nd ed. New York: W. W. Norton.
• Danielsen, Anne. 2010. “Here, There and Everywhere: Three Accounts of Pulse in D’Angelo’s ‘Left and Right.’” In Musical Rhythm in the Age of Digital Reproduction, edited by Anne Danielsen, 19–35. Burlington, VT: Ashgate.
• de Clercq, Trevor. 2012. “Sections and Successions in Successful Songs: A Prototype Approach to Form in Rock Music.” PhD dissertation, University of Rochester.
• ———. 2016. “Measuring a Measure: Absolute Time as a Factor for Determining Bar Lengths and Meter in Pop/Rock Music.” Music Theory Online 22 (3).
• de Clercq, Trevor, and David Temperley. 2011. “A Corpus Analysis of Rock Harmony.” Popular Music 30 (1): 47–70.
• Everett, Walter. 2004. “Making Sense of Rock’s Tonal Systems.” Music Theory Online 10 (4).
• ———. 2009. The Foundations of Rock: From “Blue Suede Shoes” to “Suite: Judy Blue Eyes.” Oxford: Oxford University Press.
• Fraisse, Paul. 1982. “Rhythm and Tempo.” In The Psychology of Music, edited by Diana Deutsch, 149–80. Waltham, MA: Academic Press.
• Friberg, Anders, and Andreas Sundström. 2002. “Swing Ratios and Ensemble Timing in Jazz Performance: Evidence for a Common Rhythmic Pattern.” Music Perception 19 (3): 333–49.
• Fujita, Tetsuya, Yuji Hagino, Hajime Kubo, and Goro Sato. 1989. The Beatles: Complete Scores. Milwaukee, WI: Hal Leonard.
• Hall, Anne. 2005. Studying Rhythm. 3rd ed. Upper Saddle River, NJ: Pearson Prentice Hall.
• Iyer, Vijay. 2002. “Embodied Mind, Situated Cognition, and Expressive Microtiming in African-American Music.” Music Perception 19 (3): 387–414.
• Kostka, Stefan, Dorothy Payne, and Byron Almén. 2013. Tonal Harmony with an Introduction to Twentieth-Century Music. 7th ed. New York: McGraw-Hill.
• Levy, Mark. 2011. “Improving Perceptual Tempo Estimation with Crowd-Sourced Annotations.” In Proceedings of the 12th International Society for Music Information Retrieval Conference (ISMIR), October 24–28, edited by Anssi Klapuri and Colby Leider, 317–22. Miami: University of Miami.
• London, Justin. 2012. Hearing in Time: Psychological Aspects of Musical Meter. 2nd ed. Oxford: Oxford University Press.
• ———. 2016. “Metre.” Grove Music Online. Accessed May 12, 2016. http://www.oxfordmusiconline.com/subscriber/article/grove/music/18519.
• Matthews, Neal, Jr. 1984. The Nashville Numbering System: An Aid to Playing by Ear. 2nd ed. Milwaukee, WI: Hal Leonard.
• Mattingly, Rick. 2006. All about Drums: A Fun and Simple Guide to Playing Drums. Milwaukee, WI: Hal Leonard.
• McCandless, Gregory. 2013. “Metal as a Gradual Process: Additive Rhythmic Features in the Music of Dream Theater.” Music Theory Online 19 (2).
• Moelants, Dirk. 2002. “Preferred Tempo Reconsidered.” In Proceedings of the 7th International Conference on Music Perception and Cognition (ICMPC), July 17–21, edited by Catherine Stevens, Denis Burnham, Gary McPherson, Emery Schubert, and James Renwick, 580–83. Sydney, Australia: University of New South Wales.
• Moelants, Dirk, and Martin McKinney. 2004. “Tempo Perception and Musical Content: What Makes a Piece Fast, Slow, or Temporally Ambiguous?” 8th International Conference on Music Perception and Cognition, 558–62.
• Moore, Allan. 1992. “Patterns of Harmony.” Popular Music 11 (1): 73–106.
• ———. 2001. Rock: The Primary Text: Developing a Musicology of Rock. 2nd ed. Burlington, VT: Ashgate.
• Morgenstein, Rod, and Rick Mattingly. 1997. The Drumset Musician. Milwaukee, WI: Hal Leonard.
• Osborn, Brad. 2010. “Beats That Commute: Algebraic and Kinesthetic Models for Math-Rock Grooves.” Gamut 3 (1): 43–68.
• ———. 2014. “Kid Algebra: Radiohead’s Euclidian and Maximally Even Rhythms.” Perspectives of New Music 52: 1–25.
• Parncutt, Richard. 1994. “A Perceptual Model of Pulse Salience and Metrical Accents in Musical Rhythms.” Music Perception 11 (4): 409–464.
• Pieslak, Jonathan. 2007. “Re-casting Metal: Rhythm and Meter in the Music of Meshuggah.” Music Theory Spectrum 29 (2): 219–45.
• Pollack, Alan. 1993–1999. “‘Notes On’ Series.” Accessed May 16, 2017. http://www.recmusicbeatles.com/public/files/awp/awp.html.
• Potter, Dee. 2001. The Drummer’s Guide to Shuffles. Milwaukee, WI: Hal Leonard.
• Randall, Robin, and Janice Peterson. 1997. Lead Sheet Bible. Milwaukee, WI: Hal Leonard.
• Riley, Jim. 2010. Song Charting Made Easy: A Play-Along Guide to the Nashville Number System. Milwaukee, WI: Hal Leonard.
• ———. 2015. Survival Guide for the Modern Drummer: A Crash Course in All Musical Styles for Drumset. Van Nuys, CA: Alfred Music.
• Rosenberg, Nancy. 2011. “Popular Music in the College Music Theory Class: Rhythm and Meter.” In Pop-Culture Pedagogy in the Music Classroom, edited by Nicole Biamonte, 47–71. Lanham, MD: Scarecrow Press.
• Semjen, Andras, Dirk Vorberg, and Hans-Henning Schulze. 1998. “Getting Synchronized with the Metronome: Comparisons between Phase and Period Correction.” Psychological Research 61 (1): 44–55.
• Stephenson, Ken. 2002. What to Listen for in Rock: A Stylistic Analysis. New Haven, CT: Yale University Press.
• Temperley, David. 1999. “Syncopation in Rock: A Perceptual Perspective.” Popular Music 18 (1): 19–40.
• Temperley, David, and Trevor de Clercq. 2013. “Statistical Analysis of Harmony and Melody in Rock Music.” Journal of New Music Research 42 (3): 187–204.
• Williams, Chas. 2012. The Nashville Number System. Nashville, TN: Chas Williams.
• Wyatt, Keith, Carl Schroeder, and Joe Elliott. 2005. Ear Training for the Contemporary Musician. Milwaukee, WI: Hal Leonard.
• Zoro. 2007. The Commandments of R&B Drumming: A Comprehensive Guide to Soul, Funk, and Hip-Hop. Van Nuys, CA: Alfred Music.
• ———. 2013. The Commandments of the Half-Time Shuffle: A Comprehensive Guide to the Most Beloved Yet Mystifying Grooves in Drum History. Van Nuys, CA: Alfred Music.